
The last line shows that the expression from the two lines above it are equal, indicated by the “true” on the right.Īn illustration using Desmos is here.This uses the formula from analytic geometry: The next two lines compute the inverse tangent of angle rotated counterclockwise between the segments to the foci and the normal.The “such that” bar, |, indicates that what follows it is substituted into the expression.

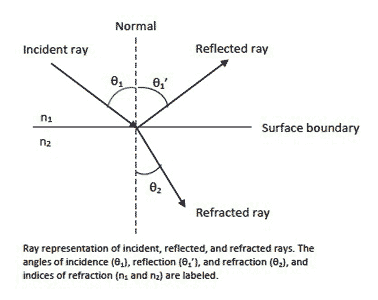
The fourth and fifth lines are the slopes, m 1 and m 3, are the slopes from the point on the ellipse to the foci.The second line finds the derivative of y and the third line, m 2 is the slope of the normal, the opposite of the reciprocal of the derivative.Solving for y there are two equations, the second one is for the upper half that we will use below. The first line starts with the ellipse with a > b > 0.The computation is done using a Computer Algebra System (CAS) and is shown below, the line-byline explanation follows: The angle of incidence and the angle of reflection are between the segments to the foci and the normal to the ellipse. The Ellipse: A light ray leaving one focus of an ellipse is reflected by the ellipse through the other focus of the ellipse. The conic sections all have interesting reflection properties that are quite useful. See the illustration of this in Desmos here and see an easier way to do this problem. To find the minimum distance find the derivative of AD + DB and set it equal to zero. Using the lengths marked in the drawing, and are all perpendicular to the total distance is AD + DB. The angle α is called the angle of incidence and the angle β is called the angle of reflection. Show that this implies that the angle α between AD and the normal to the surface is equal to the angle β between the normal and DB. In the figure below, light travels from a point A to point D on a reflecting surface CE and then to point B by the shortest total distance. Reflection is one of my favorites: show that the angle of incidence equals the angle of reflection. Nevertheless, the problems can be interesting and are useful in a practical sense. On the exams, students are given an expression and asked to find its maximum or minimum value. This step does not involve any “calculus.” If a student cannot do this or does it incorrectly, then there is no way to earn the calculus points that follow. The reason for this is that the first step is to write the equation that models the situation. While these problems are found in most of the textbooks, they almost never appear on the AP Calculus Exams. Often this is the minimum cost of producing something, or to maximize profit, or to make the largest area or volume with the least material.
Optimizations problems are situations in which some item is to be made as large or small as possible. Be careful, when assigning old questions they’re good, but they may no longer be tested.) These include the “A” topics (precalculus), Newton’s Method, work, volume by cylindrical shells among others. (Remember that the course description underwent major changes in 1998 and some topics were dropped at that time.
These may be good for your precalculus classes. Thank you Kalpana! They include precalculus topics that were tested on the exams before 1998. These were researched by by Kalpana Kanwar a teacher at Wisconsin Heights High School. An index of all free-response questions from 1971 – 2018 listed by major topics. First, a new resource has been added to the resource page.


 0 kommentar(er)
0 kommentar(er)
